Fibrecrafts – the art of knitting, crocheting, weaving, spinning, embroidering, and other related crafts – have been a lifelong passion of mine. I learned to crochet so young that I don’t remember learning. With fibrecrafts being so present in my life, it’s no surprise that I’ve learned how to bring one of my other passions – math – into it. To my delight, I’ve found that math is an excellent companion for any fibrecrafter. 

In History

Knitting has been tied to math for a long time. Codes, that favorite pastime of bored math students, have been passed through a variety of fibrecrafts, most notably knitting. We have numerous anecdotes from World War II of spies, usually women, encoding messages in their knitting. Often, these codes were based on Morse – the simplicity of Morse code lends itself well to encoding in a textile (Zarrelli, 2022). 

Spy-craft, however, is only one of the applications fibrecrafts have had. Consider the Apollo 11 mission, which landed humans on the moon for the first time. After crafting the software that the Apollo computers relied on, programmers needed to store that code in the computer’s memory. However, the working memory of computers of the day was so small that there simply wasn’t enough room to store both the software and the calculations it made. So, “rope memory” was created, wherein magnetic cords with wire woven through or around them hard-encoded the software’s ones and zeros (Brock, 2017). This rope memory was created by factory workers of the day, mostly women, who translated their experience with thread to wire. 

Circles and Spheres

In my own crochet, I’ve had to use a fair amount of math. Some of my favorite crochet is “in the round” – the term of art for crochet worked in a circle, rather than a straight row. However, circles tend to grow as one goes.  But by how much? If you’re aiming for a circle, overdoing it results in ruffles.

Whereas underdoing it causes the piece to curl up.

For people who work off of patterns, those patterns will tell you how much to increase a piece in order to keep it flat. However, I love to freehand pieces, like this blanket here. 

The magic number? Usually between six and eight. With a shorter stitch – here I’m using a single crochet – six additional stitches per row will give you a flat object. With a longer stitch – for example, a double – around eight stitches are needed. Why the difference? Well, recall that with circles, the difference in circumference between a circle of radius n and radius n+x would be 2pi*x. If x (the height of the stitch) is higher, the difference in circumference is greater, and since these stitches are all about the same width, you need more of them to make up the difference. 

Untangling Works in Progress

On a less technical note, let’s talk about yarn. Yarn is sold by weight, which might seem like an odd choice at first. After all, depending on thickness and material, 500 grams of yarn might take multiple months to work through or be used up in a single day. And, to be fair, yarns also list their yardage on the packaging – arguably more useful when you’re trying to figure out if you have enough for that scarf! 

Once you’ve made an object – let’s say half of a sweater – you have no real way to directly figure out how many yards of yarn you’ve consumed. This is a big problem if, like me, you have a tendency to misplace some of your skeins in your very messy closets. No one wants to take most of a project with them on vacation. 

So instead, you put that half-finished sweater on a scale and figure out how much it weighs – a measurement that won’t change whether the yarn is a neat ball, a work in progress, or a tangle. Since patterns come with estimates of the weight of yarn required, a little addition will make sure you never leave the last skein in your closet again. 

Pixels and Needlepoint

Finally, let’s talk about cross-stitch. Cross-stitch is a form of embroidery in which the crafter makes a series of small, cross-shaped stitches in a grid pattern in order to create a work. 

Or, to put it another way, cross-stitch is pixel art for crafters. 

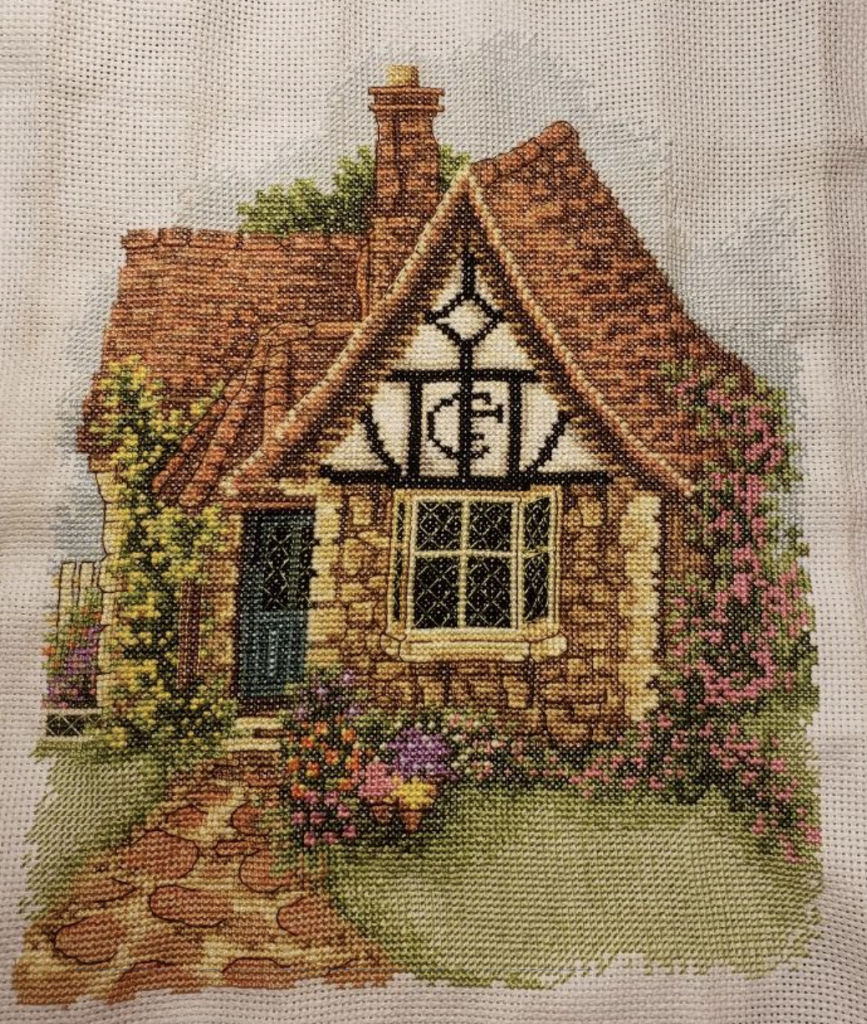
Pixelization – or converting a theoretical image into discrete units, each a single color – is rather important in computer graphics, given that pixels are how computer screens display images. Theoretically, one could cross-stitch the output of a computer screen – with a few caveats. 

First, scale. A computer screen at 1080p has just over 2 million individual pixels. On a denser cross-stitch, at 18 stitches per inch, that cross-stitch would end up at almost 9 by 5 feet – a fairly big thing to hang on a wall. And, assuming one stitch per second, that cross-stitch would take 72 eight-hour shifts to complete. 

Second, cross-stitches have to contend with the limited number of colors available to them. While computer screens can usually display over 16 million colors (EIZO, 2009), crafters are limited to the number of colors produced by modern floss companies. One of the giants of floss production in America, DMC, produces a mere 506 colors in its cotton embroidery floss line, conveniently numbered between B5200 (Snow White) and 150 (Dusty Rose Ult Vy Dk) (Jackie, 2020). With such a limited range of colors, computer algorithms that aim to match hues as closely as possible may end up, for example, placing a blue-purple immediately next to a red-purple, in an attempt to capture a smooth gradient of a medium purple. 

Because of these limitations, while cross-stitch conversion programs abound on the internet, experienced crafters (myself included!) still spend the money for patterns that have been edited and tested by actual humans. Those actual humans, however, often start with the output of a conversion program – so all of this technology (and math) saves them a ton of time. 

In Conclusion

With all of these connections, fibrecrafts and math reveal themselves to be a natural pairing. If you’ve never picked up a fibrecraft, but you’re a big fan of math, consider giving it a try. And if you prefer crochet to calculus, maybe it’s time to dust off those old geometry skills. Either way, keep in mind: arts and maths aren’t as far apart as we might think.

Columnist: Skyler Matuska